


Next: Function values at particular
Up: Representing numerical results
Previous: The global representation
In order to get a more detailed impression of the quality of a solution,
one can show graphs of the function
 in the following one-dimensional subsets of
in the following one-dimensional subsets of
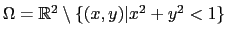 . The following choices seem most relevant:
. The following choices seem most relevant:
- Along fixed vertical cuts
to get an impression of the global behaviour.
- Along fixed horizontal cuts
to get an impression of the global behaviour.
- Along
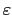 -dependent radial cuts
-dependent radial cuts
to get an impression of the boundary layer behaviour.
- Along
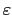 -dependent horizontal cuts
-dependent horizontal cuts
to get an impression of the interior layer behaviour.
Figure 8:
The location of the cuts in the 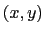 -plane.
-plane.
Horizontal cuts along
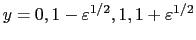 ,
vertical cuts at 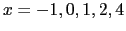 and radial cuts at
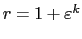 ,
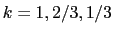 .
|
As an example, in the Figures 9, 10 11 and 12
we show results for these cuts for the case
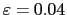 .
.
Figure 9:
The solution
 along vertical cuts in the
along vertical cuts in the 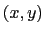 -plane.
-plane.
|
Figure 10:
The solution
 along radial cuts in the
along radial cuts in the 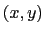 -plane.
-plane.
|
Figure 11:
The solution
 along horizontal cuts
along horizontal cuts
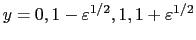 in the
in the 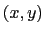 -plane.
-plane.
|
Figure 12:
The solution
 along horizontal cuts
along horizontal cuts
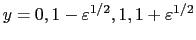 in the
in the 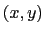 -plane.
-plane.
|



Next: Function values at particular
Up: Representing numerical results
Previous: The global representation
![]() in the following one-dimensional subsets of
in the following one-dimensional subsets of
![]() . The following choices seem most relevant:
. The following choices seem most relevant:
![]() .
.